Basics – Foldable Aquifer Project

November 27, 2019November 28, 2019

Groundwater Storage

Objectives: Explore the relationship between confined and unconfined aquifer storage properties.

1. Groundwater storage is defined by the term storativity ( or storage coefficient), which represents the volume of water released from an unit area aquifer due to a unit drop in head. This can be thought of as the volume of water (m³) drained from an aquifer that has an areal extent of one square meter and a decline of head of one meter. However, the mechanisms for this draining of the aquifer a different for a unconfined aquifer as compared to a confined aquifer. As a result, we have to sub-terms for which specific yield describes storage in a unconfined aquifer and specific storage describes storage in a confined aquifer. Below is the equation that relates these two sub-terms to storativity:

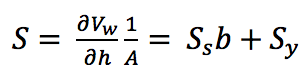
Where:

V_w is the volume of water released form the aquifer [L³]

h is the hydraulic head [L]

A is the areal area of the aquifer you are evaluating [L²]

S_s is the specific storage [1/L]

b is the aquifer thickness [L]

S_y is the specific yield [-]

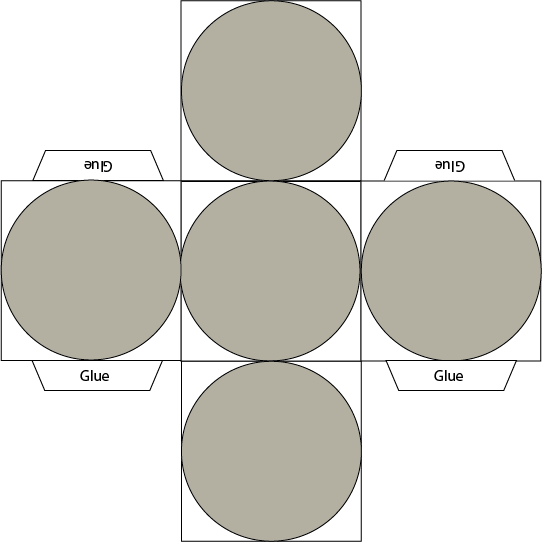
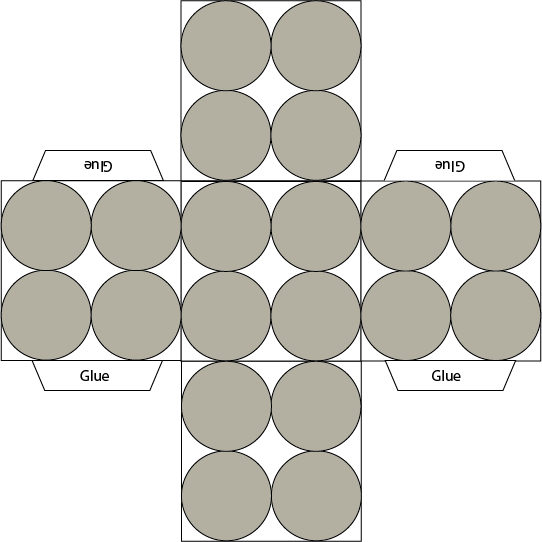
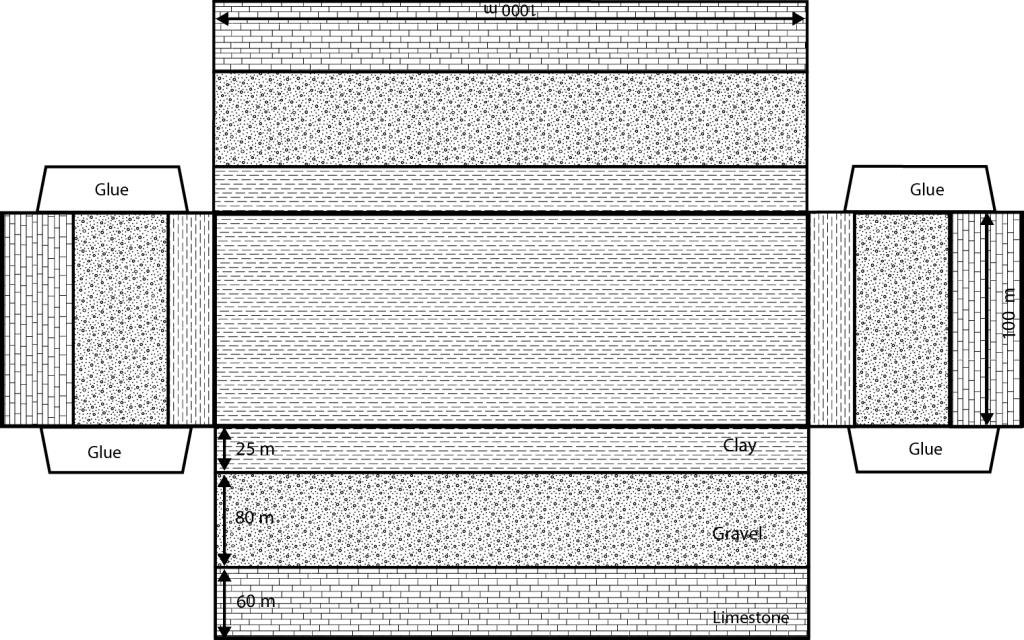
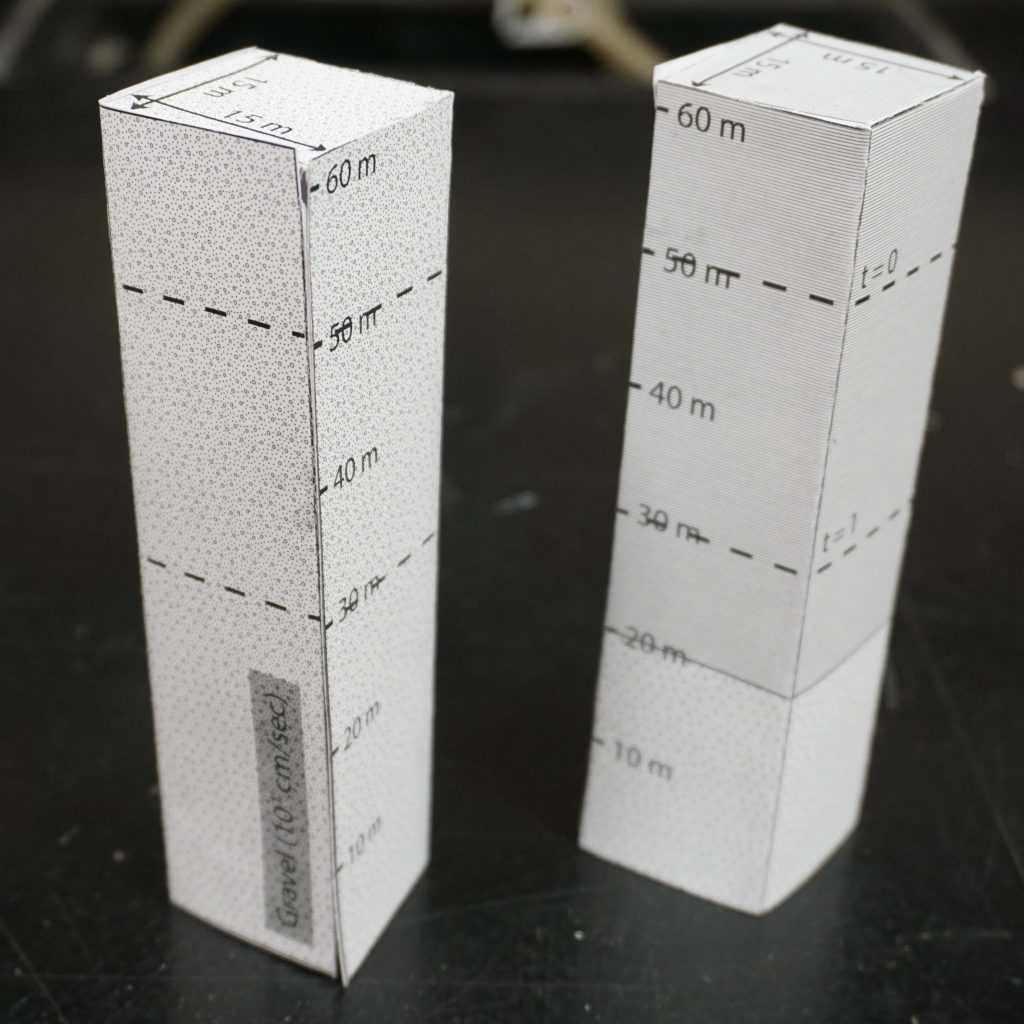
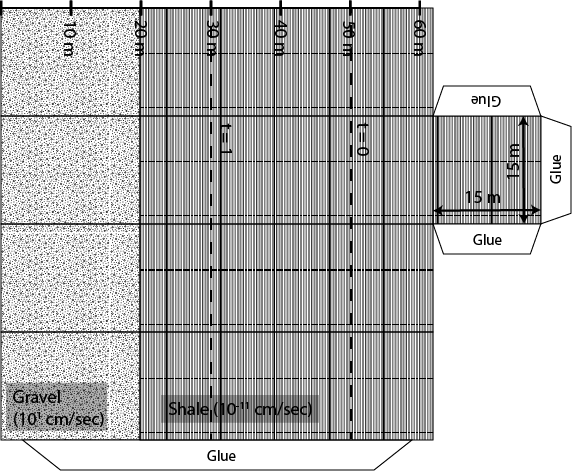
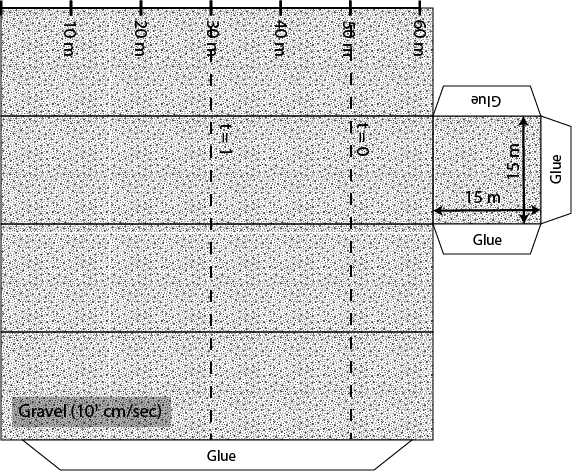
Using the foldable aquifer models given below answer the following questions assuming that the volume of water released in each of the aquifers is 945 m³.

A. Quantify the specific yield in the unconfined aquifer.

B. Quantify the specific storage in the confined aquifer.

C. Describe the difference between the storage mechanism in the unconfined aquifer as compared to the confined aquifer.

Foldable Aquifer Model – Ss Download

Foldable Aquifer Model – Sy Download

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.

November 27, 2019February 14, 2020

Porosity and Grain Packing

Objectives: Determine the relationship between grain packing and porosity of an aquifer.
1. Porosity describes the percentage of void space in a cubic unit (volume) of an aquifer. This porosity is controlled by the packing (geometry) of grains. In this problem we are going to explore the simplest of packing: Cubic Packing. As a warm up we will first look at the porosity of a single grain of sand inside a cubic value of aquifer. The second part of this problem will explore cubic packing with eight grains inside a square volume of aquifer. Use the foldable aquifer model provided to answer the following questions.

A. Determine the porosity of the cubic aquifer with a single spherical grain.

B. Determine the porosity of an aquifer with cubic packing.

C. Explain the relationship between the porosity of the single spherical grain model and the cubic pacing model with eight grains.

Foldable Aquifer Model Download

Foldable Aquifer Model Download

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.

October 27, 2019November 28, 2019

Example Foldable Aquifer Model

The goal of these paper aquifer models is to enable the user to easily visualize the three dimensional nature of groundwater related problems. For many problems it is helpful to have a physical model that one can hold in order to better understand the problems being asked. The hope is that these paper aquifer models will clarify concepts in groundwater science. This brings up a relevant quote by statistician George Box:

“Essentially, all models are wrong, but some are useful” – Box

The hope here is that these models will be useful in understanding groundwater flow in three dimensions.

Each of the paper aquifers in this set of examples will need to be constructed by the user and are sized to be printed on a standard sheet of paper. After printing the aquifer it is necessary to cut along the outer most edge of the model. Then fold each of the outer panels along the solid lines. This should form a three dimensional box or polygon. The example show below will result in a rectangular box. Each model has tabs or wings on the outer edge, which are meant to have glue or tape applied to help hold the model together. A representation of one of these models is show below along with a link to download a PDF version to experiment with.

Foldable Aquifer Model Download

Each paper model has a specific lithology (geologic layering) which for future calculation will have an associated hydraulic conductivity. As a reminder, hydraulic conductivity is simply a measure of the resistance to flow of water in a rock (it is a lot like resistance to the flow of electrical current in a circuit). The paper model also has lines with arrows showing the length, width, and height of the lithologic layers. At times and for specific problems there will be the need to apply a vertical exaggeration to these models. Pay special attention to the stated dimensions of these models because they will have to change from one problem to the next.
I would encourage the user to give this example model a try. It is a quick model to construct and it will provide some experience as to how to construct future models in this project.

Side note: It should be stated that these are models and models are not perfect. There will be times in working some of the upcoming problems where the user will need to use a bit more of their imagination to fully visualize the problem.

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.